
5.2 Atmospheric Correction of Remotely Sensed Data

5.2 Atmospheric Correction of Remotely Sensed Data
Atmospheric correction is a major issue in visible or near-infrared remote sensing because the presence of the atmosphere always influences the radiation from the ground to the sensor.
The radiance that reaches a sensor can be determined by
Normally Lmax, Lmin and DNrange are known from the sensor manufacturer or operator.
However, Ls is composed of contributions from the target, background and the atmosphere (Figure 5.5):
As introduced before, the atmosphere has severe effects on the visible and near-infrared radiance. First, it modifies the spectral and spatial distribution of the radiation incident on the surface. Second, radiance being reflected is attenuated. Third, atmospheric scattered radiance, called path radiance, is added to the transmitted radiance.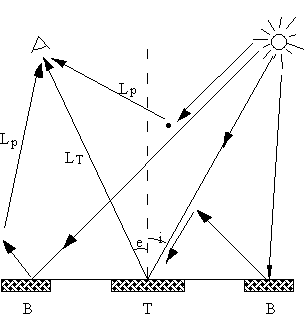
Figure 5.5 Target, background and scattered radiation received by the sensor.
Assuming that Ls is the radiance received by a sensor, it can be divided into LT and LP
LS = LT + LP (1)For a given spectral interval, the solar irradiance reaching the earth's surface isLT is the transmitted radiance.
LP is atmospheric path radiance.
Obviously, our interest is to determine LT.
EG
=
Surface can be either specular or diffuse. Most surfaces can be considered as approximate diffuse reflectors at high solar elevations, i.e. when i is small.where ES is the solar irradiance outside the atmosphere,
Ti atmospheric transmittance along the the incident direction,i incident angle
Ed diffuse sky irradiance
If the surface is assumed to be a perfect
diffuse reflector i.e. the Lambertian case, the ratio of the radiation
reflected in the viewing direction to the total radiation into the whole
upper hemisphere is given by
.
Based on Lambertian assumption,
where
is the target reflectance, Te is the transmittance
along the viewing direction. Therefore in order to quantitatively analyze
remotely sensed data, i.e. to find r,
atmospheric transmittance T and path radiance Lp
have to be known.
5.2.1
Single scattering atmospheric correction
In practice, (2) and (3) can be written
as

Path radiance LpLp is determined by at least two parameters: single scattering albedo and single scattering phase function.
Single scattering albedo = 1 when no attenuation occurs. Single scattering phase function denotes the fraction of radiation which is scattered from its initial forward direction to some other direction.
For Rayleigh atmosphereFrom the above diagram, it can be seen that forward scattering is dominated by aerosols while back scattering is mainly due to Rayleigh scattering.
For Mie's atmosphere

A number of path radiance determination algorithms exists. For a nadir view as Landsat MSS, TM and SPOT HRV are usually used. Lp for these algorithms can be determined by:

P is a combination of Mie and Ragleigh atmosphere.For aerosol scattering, the phase function Pp(µi) does not change much as wavelength changes, the function for l = 0.7 mm can be used for all wavelengths. This function is usually found in a diagram or a table form. See a function found in Forster (1984).
The average background B is usually determined by collecting ground-truth information for a region. A 3 km x 3 km square centering the pixel to be corrected can be used.
Sky Irradiance and Ground Irradiance

In this section, we only tried to introduce some basic concepts of this complex topic. This is only a single-scattering correction algorithm for nadir viewing condition. More sophisticated algorithms which counts multiple-scattering do exist. Some examples of these algorithms are LOWTRAN 7, 5S (Simulation of the Satellite Signal in the Solar Spectrum 5S) and 6S (Second Simulation - aircraft, altitude of target). There are FORTRAN codes available for these algorithms. The 5S and 6S are proposed by Tanre and his colleagues (e.g. Tanre et al., 1990, IGARSS 190, p. 187).
One has to be careful when conducting atmospheric correction since there are many factors to be counted and to be estimated. If these estimations are not properly made, the atmospheric correction might add more bias than does the atmosphere itself.
5.2.2 Dark-target atmospheric correction
This is most suitable to the clear sky when Rayleigh atmosphere dominates since Rayleigh scattering affects short wavelength, particularly visible, and we know that clear-deep water has a very low spectral reflectance in the short wavelength region. If a relatively large water body, say 1-2 km in diameter, can be found on an image, we can use the radiance of water derived from the image as Lw and the real water radiance, L, to estimate Lp.
Lw = K ï DN water + LminFor the infrared channels, Rayleigh atmosphere has little effects and Lp is assumed to be 0. It can be seen that this method only applies to Rayleigh atmosphere.Lp = Lw - L
Lp can then be subtracted from other radiances in an image for the visible channels.
5.2.3 Direct digital number to reflectance transformation
This can be done by
R = a ï DN + b
![]()